Can You Trek The Triangle?

Riddler Classic

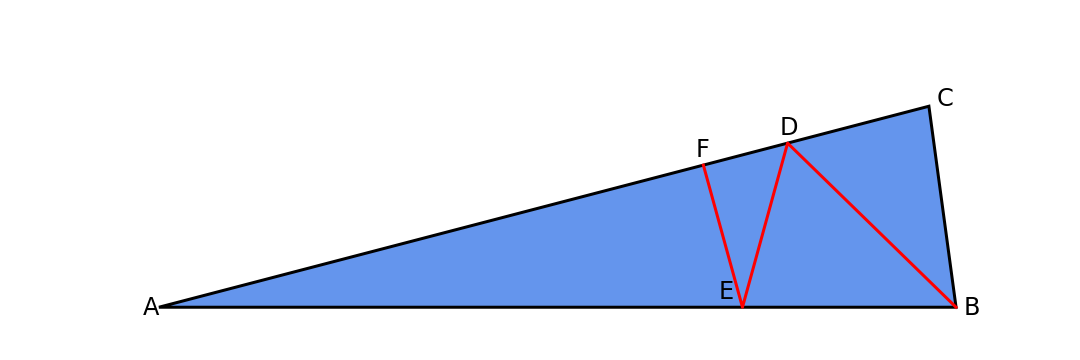
Amare the ant is traveling within Triangle ABC, as shown below. Angle A measures 15 degrees, and sides AB and AC both have length 1.

Amare starts at point B and wants to ultimately arrive on side AC. However, the queen of his colony has asked him to make several stops along the way. Specifically, his path must:

• Start at point B.
• Second, touch a point — any point — on side AC.
• Third, touch a point — any point — back on side AB.
• Finally, proceed to a point — any point — on side AC (not necessarily the same point he touched earlier).

What is the shortest distance Amare can travel to complete the queen’s desired path?

Solution

Let $BF$ be the shortest route for Amare. Unfolding the triangle yields the following shape.

Answer

$\angle C'AB = \angle ABF = 45^o$. Therefore $BF = \frac{1}{\sqrt{2}}$. The path looks like this.

The following angles provide "directions" for Amare.
$\angle ABD = 45^o$
$\angle ADE = 45^o + 15^o = 60^o$
$\angle AEF = 60^o + 15^o = 75^o$

Rohan Lewis

2022.01.10

Code can be found here.