Solution¶
Given voting population $p$, Let $\dfrac{A_e}{p}$, $\dfrac{B_e}{p}$, $\dfrac{A_m}{p}$, and $\dfrac{B_m}{p}$ represent the percent of people who vote for candidates $A$ and $B$, either on election night or by mail.
We know the following:
- $1 = \dfrac{A_e}{p} + \dfrac{B_e}{p} + \dfrac{A_m}{p} + \dfrac{B_m}{p}$
- $0.8 = \dfrac{A_e}{p} + \dfrac{B_e}{p}$
- $0.2 = \dfrac{A_m}{p} + \dfrac{B_m}{p}$
Since the people vote randomly and independently, $\dfrac{A_e}{p}$ has a frequency distribution that is binomial.
$μ = 0.8 \cdot \frac{1}{2} = 0.4 \textrm{ or } 40\%$
$σ = \sqrt{0.8 \cdot \frac{1}{2} \cdot \frac{1}{2}} = \sqrt{0.2} \approx 4.47\%$
Here are three cases to examine.
Case 1 : Mail In Votes are Irrelevant, $A$ Wins¶
$\dfrac{A_e}{p} - \dfrac{B_e}{p} > 20\%$
It follows that $\dfrac{A_e}{p} > 50\%$ and $\dfrac{B_e}{p} < 30\%$. This accounts for $1.267\%$ of the election results.
Case 2 : Mail In Votes are Irrelevant, $B$ Wins¶
$\dfrac{B_e}{p} - \dfrac{A_e}{p} > 20\%$
It follows that $\dfrac{B_e}{p} > 50\%$ and $\dfrac{A_e}{p} < 30\%$. This accounts for $1.267\%$ of the election results.
Case 3 : At Least One Mail In Vote Is Necessary¶
$\left| \dfrac{A_e}{p} - \dfrac{B_e}{p} \right| \le 20\%$
$97.46\%$ of the election results fall in this case.
The follow chart summarizes the results. $\%$ is in terms of $\dfrac{A_e}{p} - \dfrac{B_e}{p}$ for Election Night Vote and $\dfrac{A_m}{p} - \dfrac{B_m}{p}$ for Early Vote by Mail.
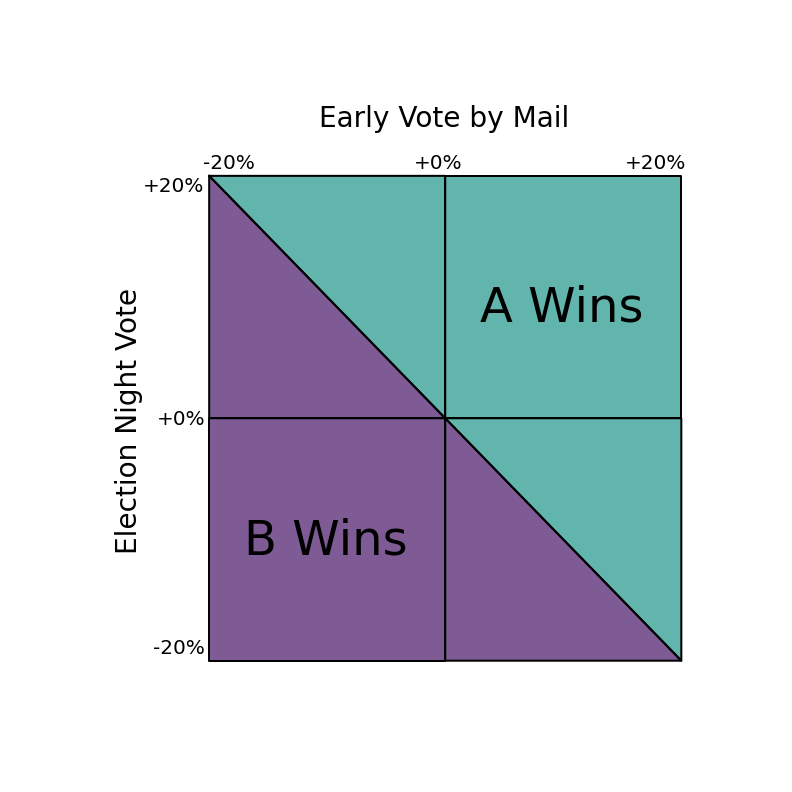
Note that if Candidate A had fewer votes on Election Night (bottom row), they win $25\%$ of the time. Similary, if Candidate B had fewer votes on Election Night (top row), they win $25\%$ of the time.
Answer¶
$97.46\% * 25\% = 24.37\%$
Rohan Lewis¶
2021.04.26¶
Code can be found here.