Can You Cut The Square…Into More Squares?¶

Riddler Express ¶

From Rob Peacock comes a matter of squaring a multitude of squares:

There are many ways to slice a big square into smaller squares (not necessarily of equal size), so that the smaller squares don’t overlap, while still making up the entire area of the big square.

For example, you can slice the big square into four smaller squares, each a quarter of the area of the big square. Or you could slice it into seven squares, if you take one of those four squares and slice it into four yet smaller squares.

What whole numbers of squares can you not slice the big square into?

Solution¶

Another useful slicing for more whole numbers is slicing the big square into $n^2$ squares, and then recombining $(n-1)^2$ squares into a single square.

Some examples are shown below:

$$n^2 - (n-1)^2 + 1$$

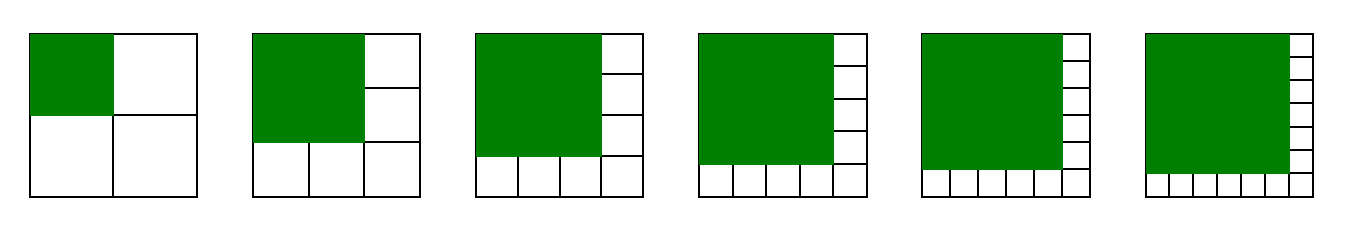
$$= 2n$$
$$= 4,6,8,...$$

Any 1 square from the above set can be divided into 4 squares, yielding $7,9,11,...$, as illustrated below:

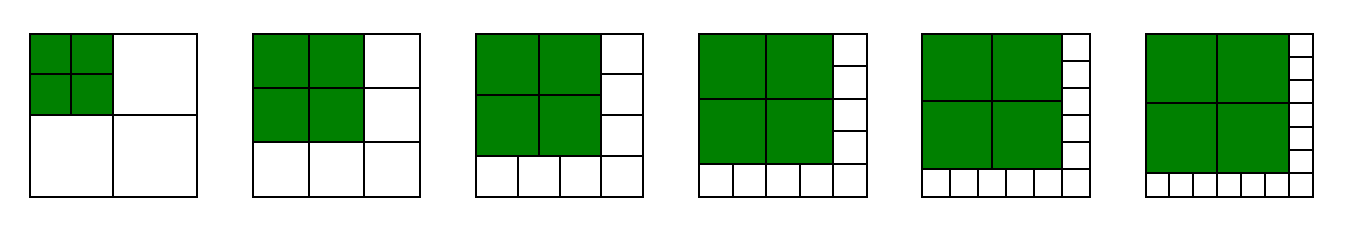
Answer¶

2, 3, and 5 are the only impossible solutions.

Rohan Lewis¶

2021.01.10¶